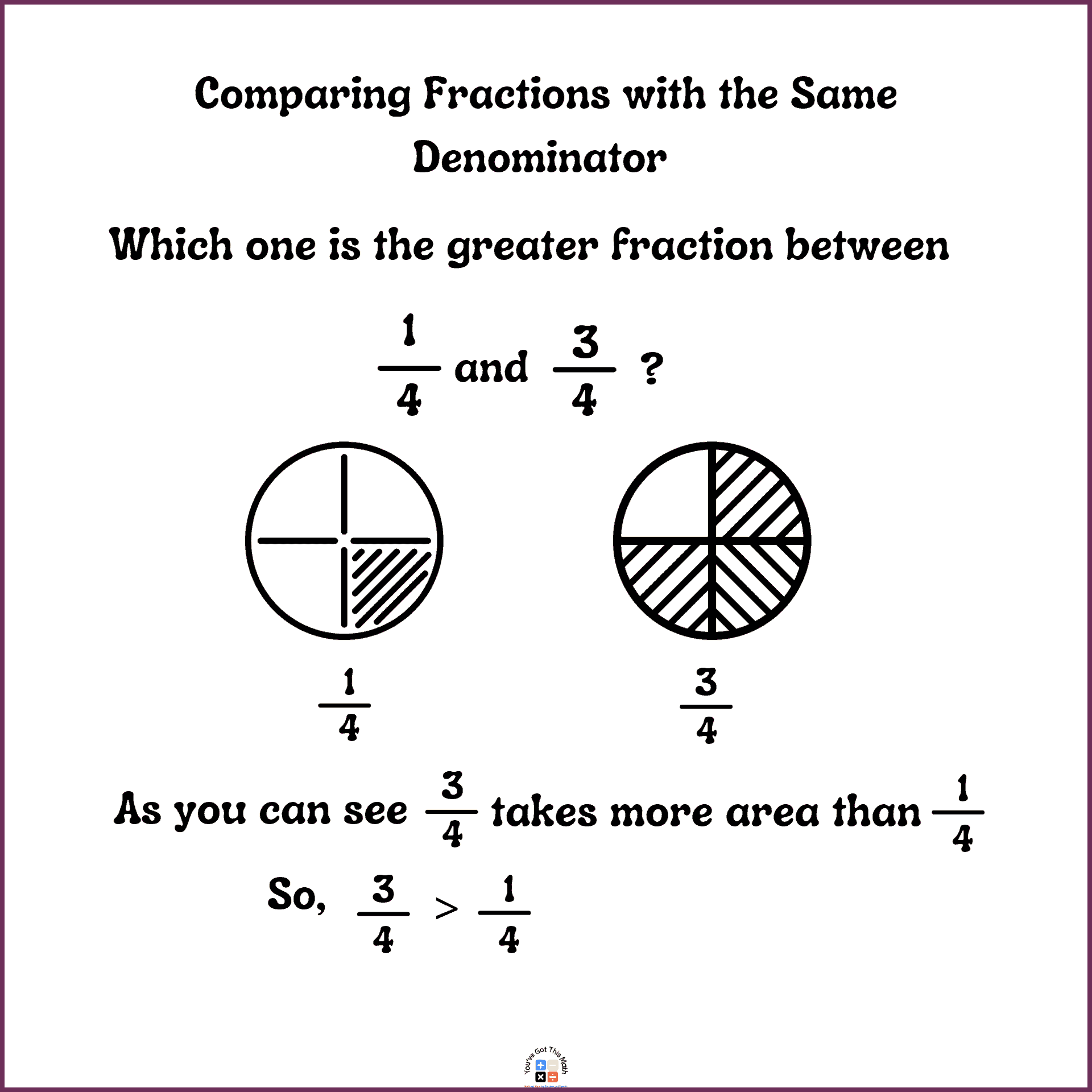6 Free Compare Fractions with the Same Numerator Worksheets with Answer Key
These compare fractions with the same numerator worksheets will help to visualize and understand fractions and parts of fractions. 3rd and 4th grade students will learn basic comparing methods and can improve their basic math skills with our free printable compare fractions with the same numerator worksheets.
6 Worksheets to Compare Fractions with the Same Numerator
With the aid of these worksheets, our kids will be able to master fundamental mathematics quickly and actively. Download the following worksheets and practice.
The numerators and denominators are the basic portions of fractions. If you want to have some mathematical jobs to do with fractions, you need to know the numerators and denominators. Stay with us in this article to have a firm knowledge about all of these.
Follow the detailed instructions provided below. You can also arrange interactive games using these activities to make learning more effective.
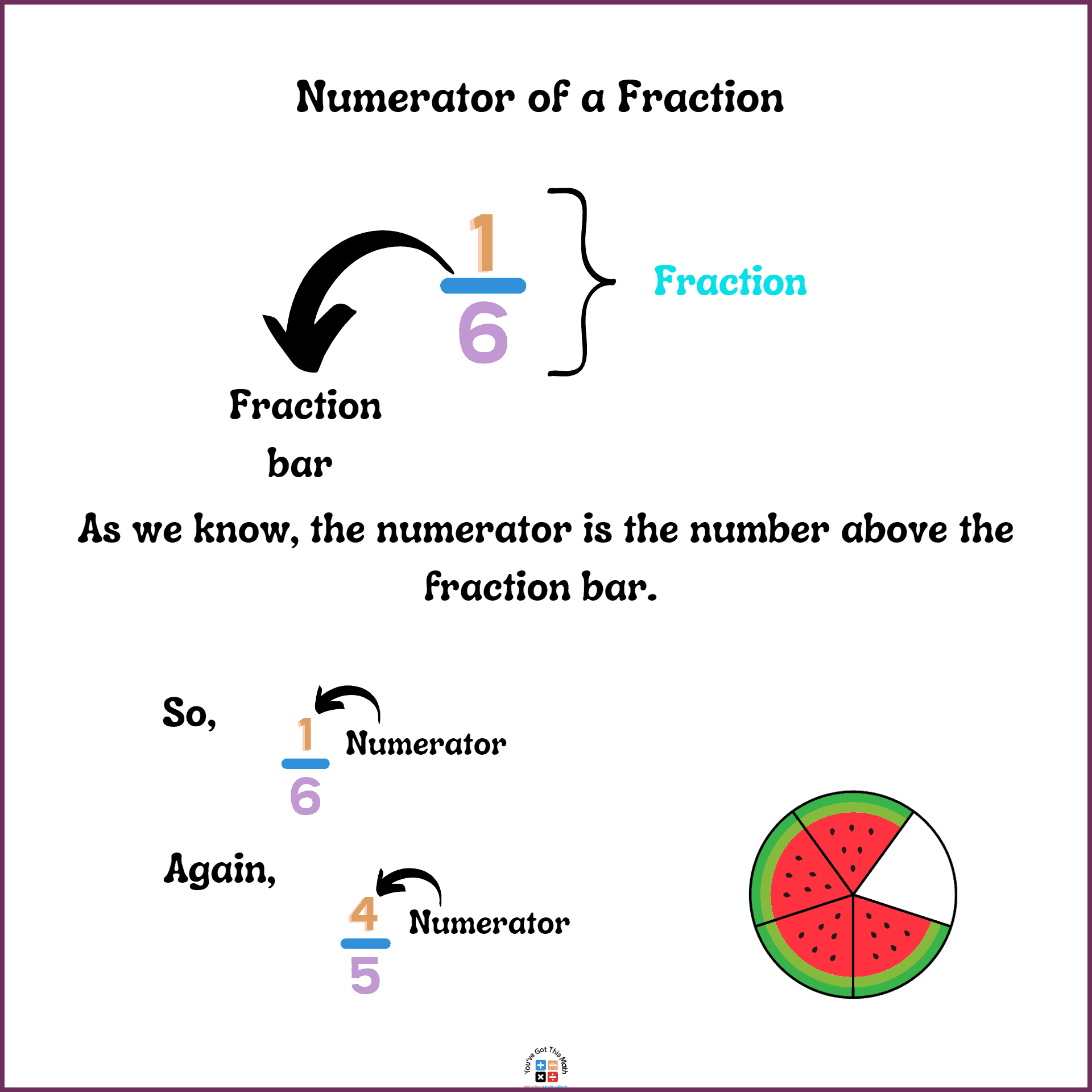
Introduction to Numerator of a Fraction
A fraction consists of two numbers printed one above the other and separated by a fraction bar, which is a horizontal line. The numerator is the number above the fraction bar, while the denominator is the number below the fraction bar.
For instance, if you take a fraction a/b, a is the numerator and b is the denominator. If we take the numbers as nominators and denominators like- ⅘ then 5 is the denominator and 4 is the nominator here.
Here, we are going to describe 5 easy ways to compare fractions with the same numerator. This is a very basic mathematical operation regarding fractions.
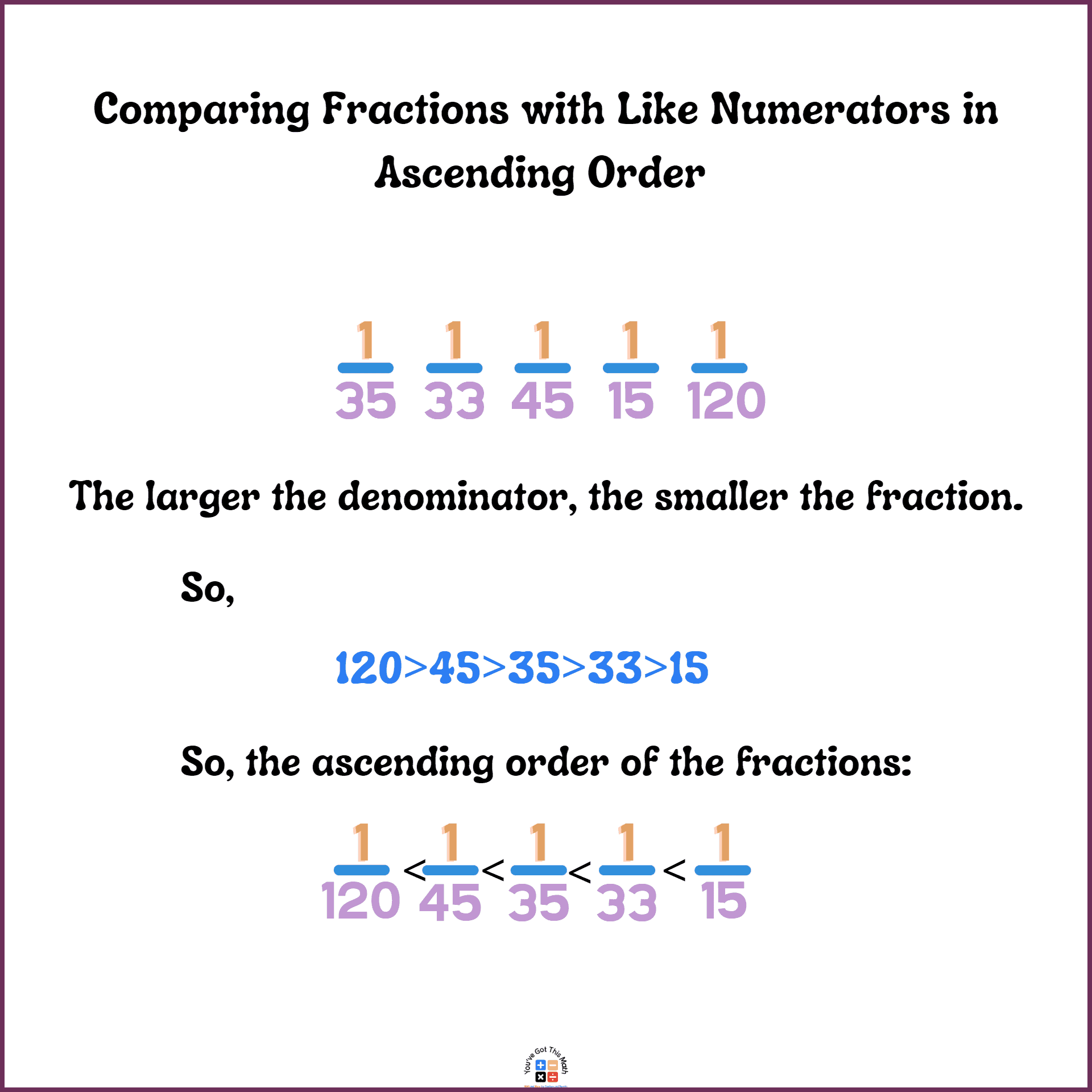
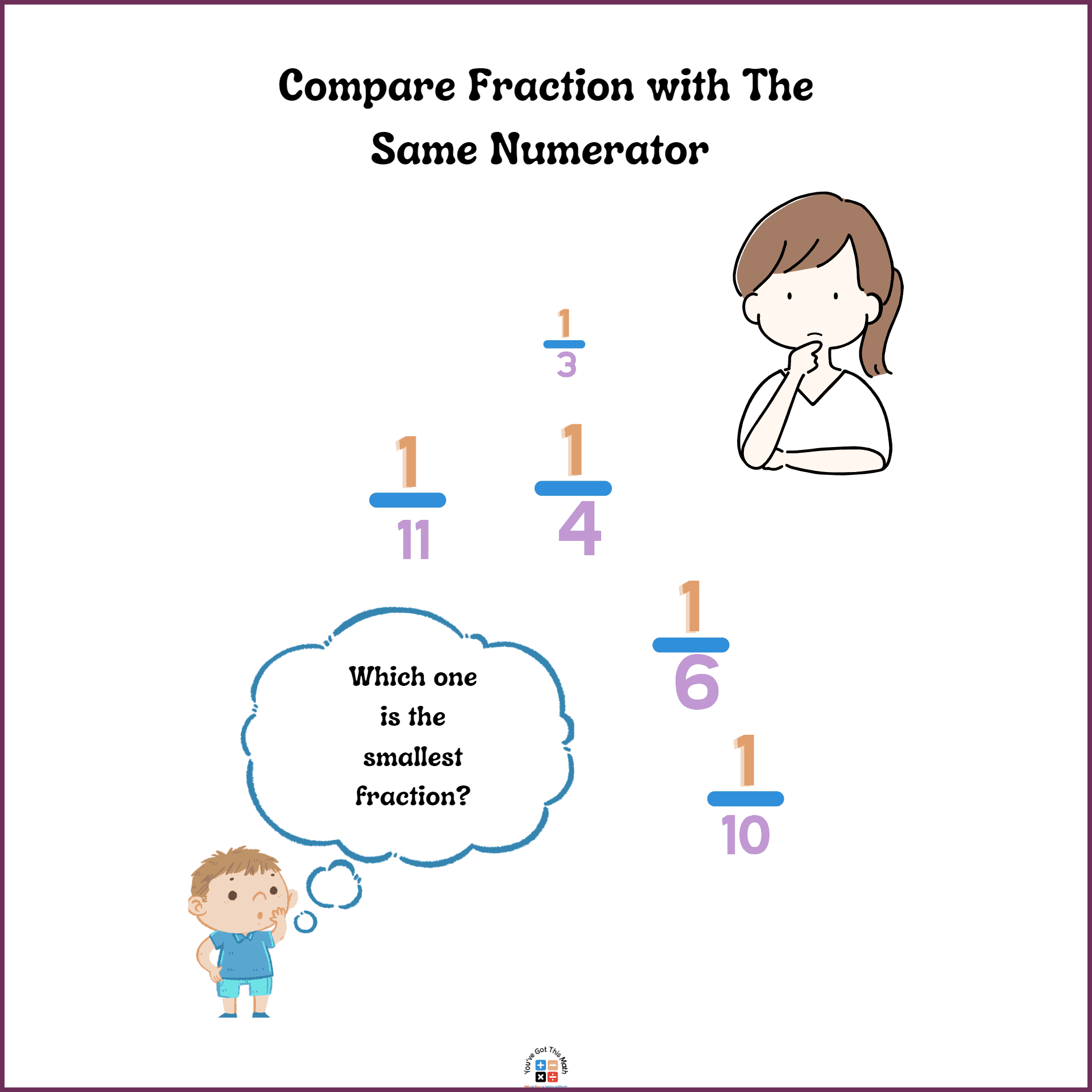
Comparing Fractions with Like Numerators in Ascending Order
Ascending order is a method of ordering numbers from smaller to bigger. Ascending means increasing. If you have a set of numbers 2, 3, 5, 9, 6, and 1, the ascending order for the numbers will be 1<2<3<5<6<9. If you have some fractions with the same numerator and need to make an ascending order, it is quite an easy task. The main idea of this activity: the larger the denominator, the smaller the fraction.
Let’s practice the above method in the following worksheet.
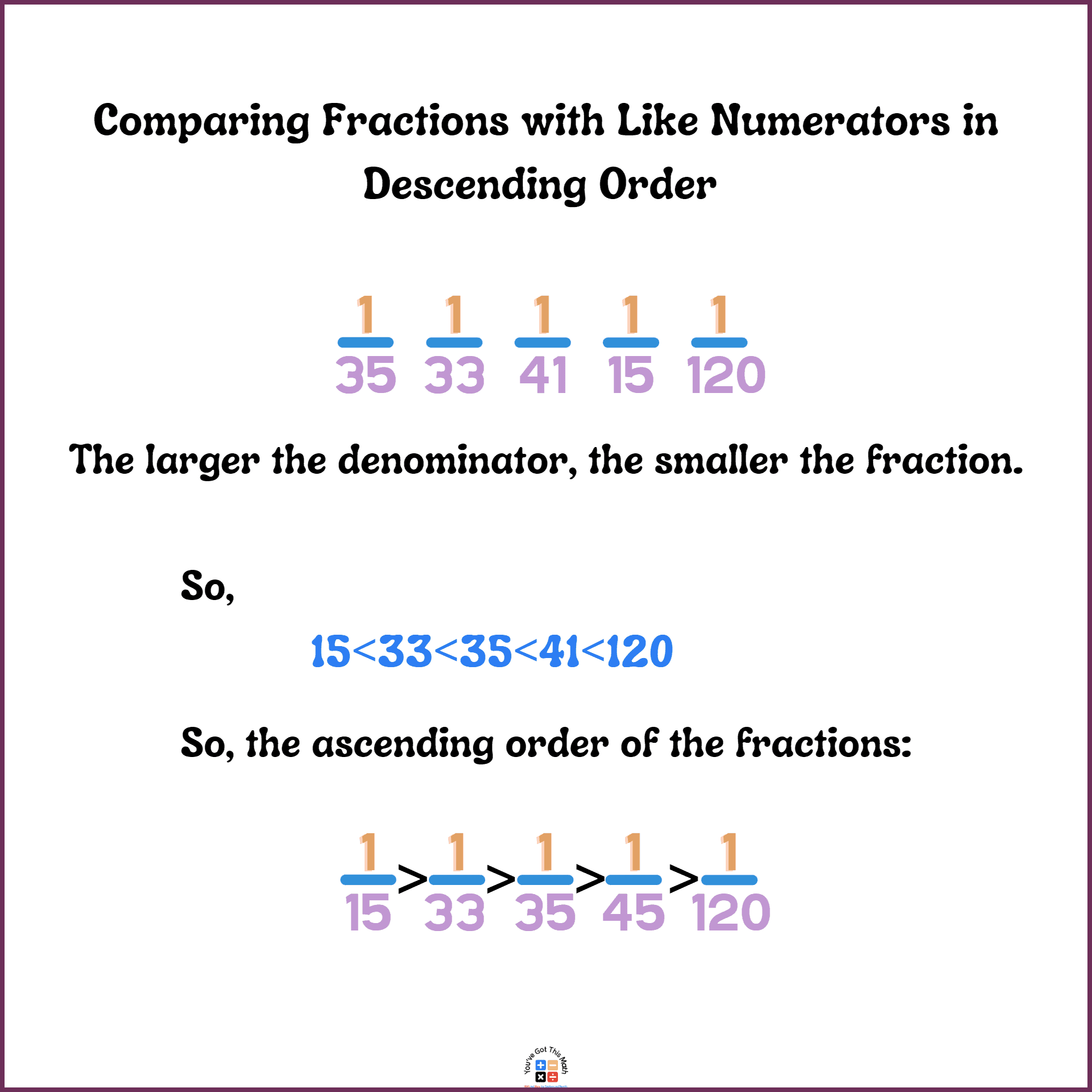
Comparing Fractions with the Same Numerators in Descending Order
Descending order is a method of ordering numbers from bigger to smaller. Ascending means decreasing. If you have a set of numbers 4, 3, 5, 6, 9, and 7, the descending order for the numbers will be 9>7>6>5>4>3. If you have some fractions with the same numerator and need to make a descending order, it is quite an easy task. The main idea of this activity: the smaller the denominator, the larger the fraction.
Try out the aforementioned technique using the worksheet that follows.
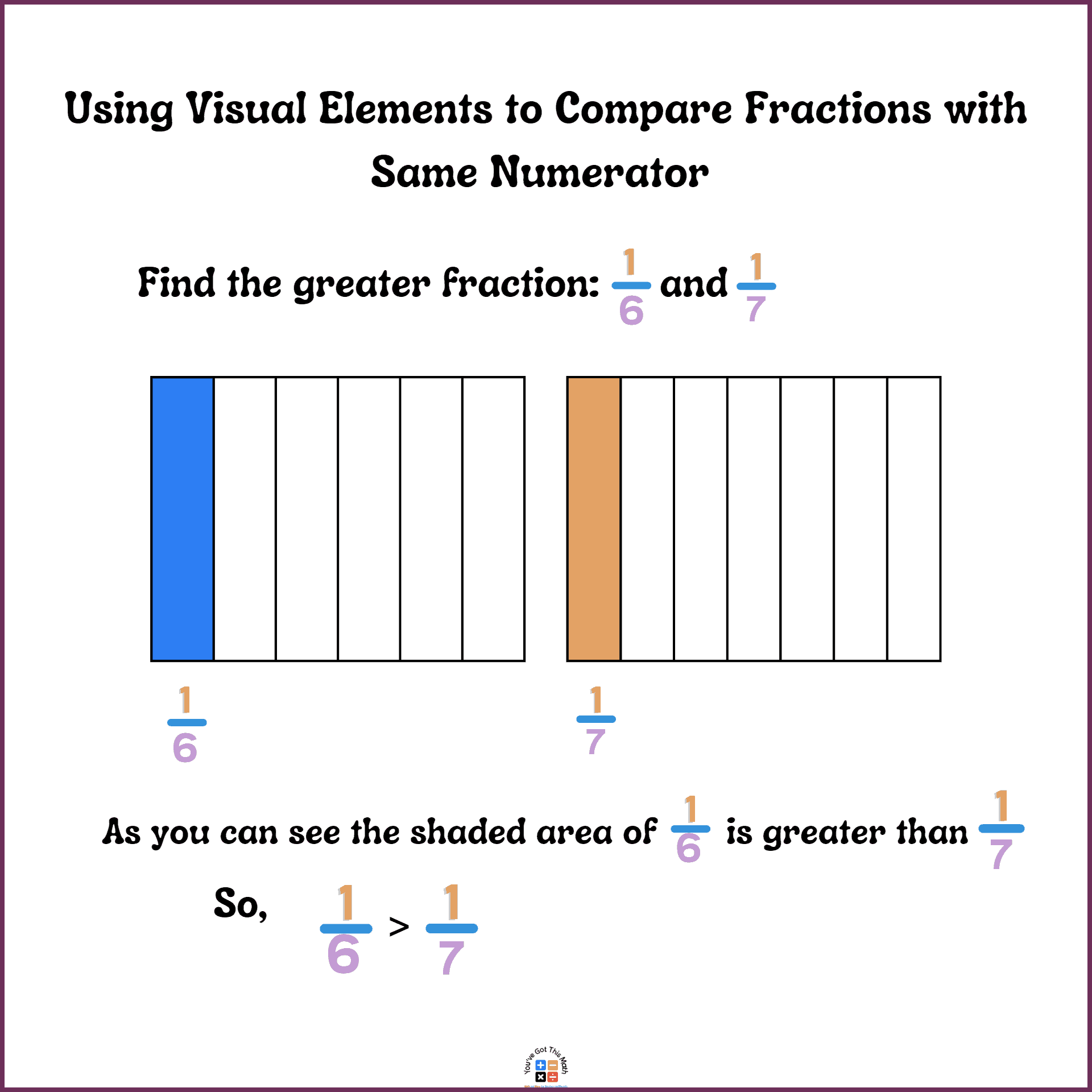
Using Different Types of Visual Elements to Compare Fractions with the Same Numerator
In this portion, I will use different types of visual elements to compare fractions. We can use squares, rectangles, circles, and triangles to represent fractions. Let’s see an example of visual elements to compare fractions.
Try out the technique mentioned above using the worksheet that follows.
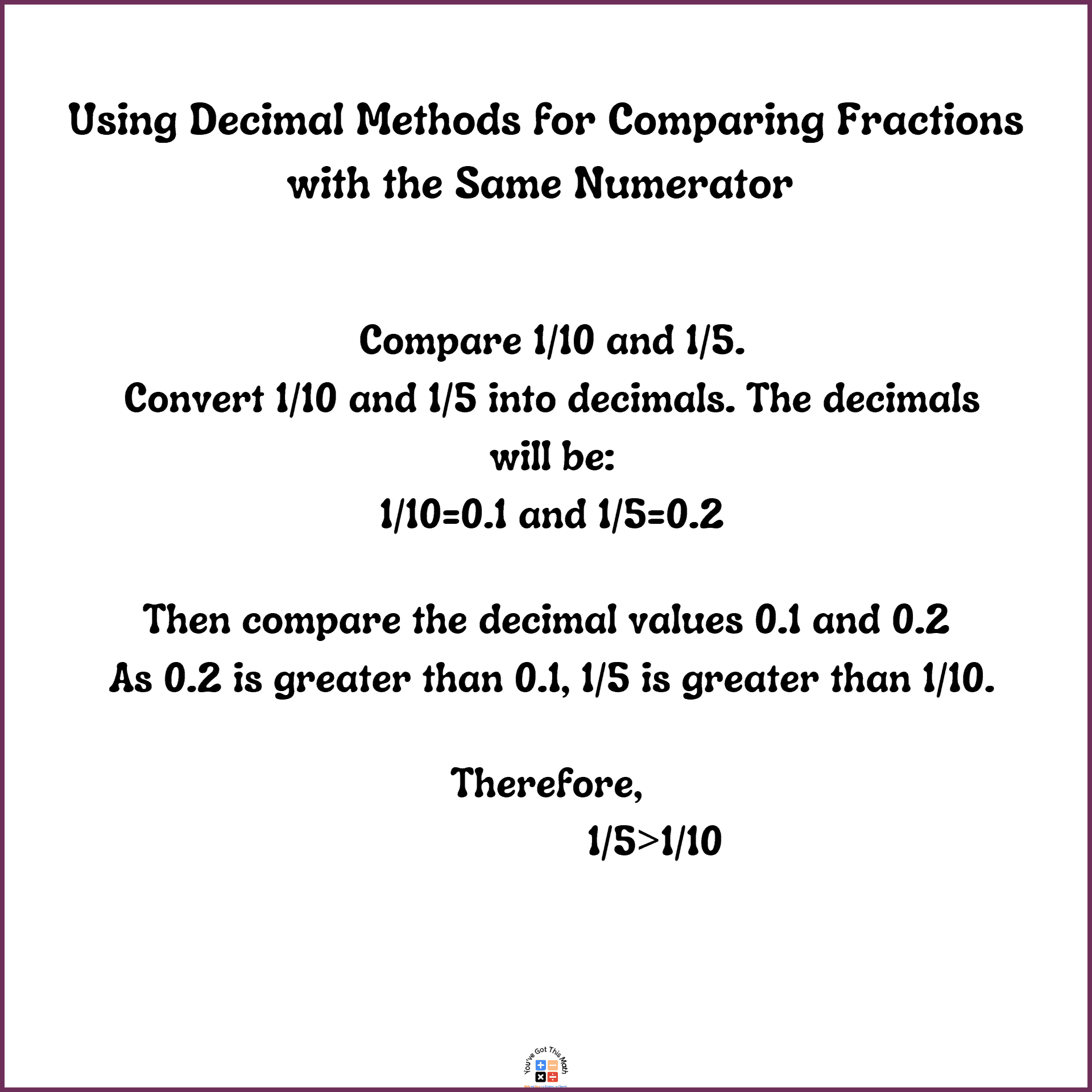
Comparing Fractions with the Same Numerator by Using Decimal Method
By using decimal procedures, you may compare fractions. Here, we contrast fractions’ decimal values. In this case, the fraction is transformed into decimals by dividing the numerator by the denominator. The decimal values are then contrasted.
Let’s follow the following worksheet to practice more.
Using Cross Multiplication to Compare Fractions with the Same Numerator
We multiply the numerator of one fraction by the denominator of the second fraction to compare fractions using cross multiplication. Let’s use an illustration to better grasp this. Compared to 1/4, 1/6. See the illustration below for a clearer explanation of this.
Try out the aforementioned technique using the worksheet that follows.
Comparing Fractions with the Same Numerator and Denominator
We have discussed how to compare fractions with the same numerator in the former sections of this article. Now I will show how to compare fractions with the same denominators. Let’s take two fractions ¼ and ¾ as examples. Here, you should bear in mind that for the same denominator, the greater the numerator, the greater the fractions. As in the given fraction, 3>1. Then ¾>¼.
Follow the procedure and practice in the worksheet attached with the article.
Download the Free Worksheet PDF
Download the following free worksheet and practice with your students.
So today, we’ve discussed comparing fractions with the same numerator worksheets using the concepts of comparing fractions in ascending orders and descending orders, using visual elements, decimal method, and cross multiplication. Download our free worksheets, and after practicing these worksheets, students will surely improve their mathematical skills and have a better understanding of fractions.

Hi there! This is Souptik Roy, a graduate of the Bangladesh University of Engineering and Technology, working as a Content Developer for the You Have Got This Math project of SOFTEKO. I am a person with a curious and creative mind. After finishing my Engineering degree, I want to explore different fields. This is why I am working here as a content developer. I have a massive interest in creative content writing. When I find that someone can learn something from my articles, this gives a lot of inspiration. hopefully, you will find interest in my article, if you have a child and want to teach them math with fun.