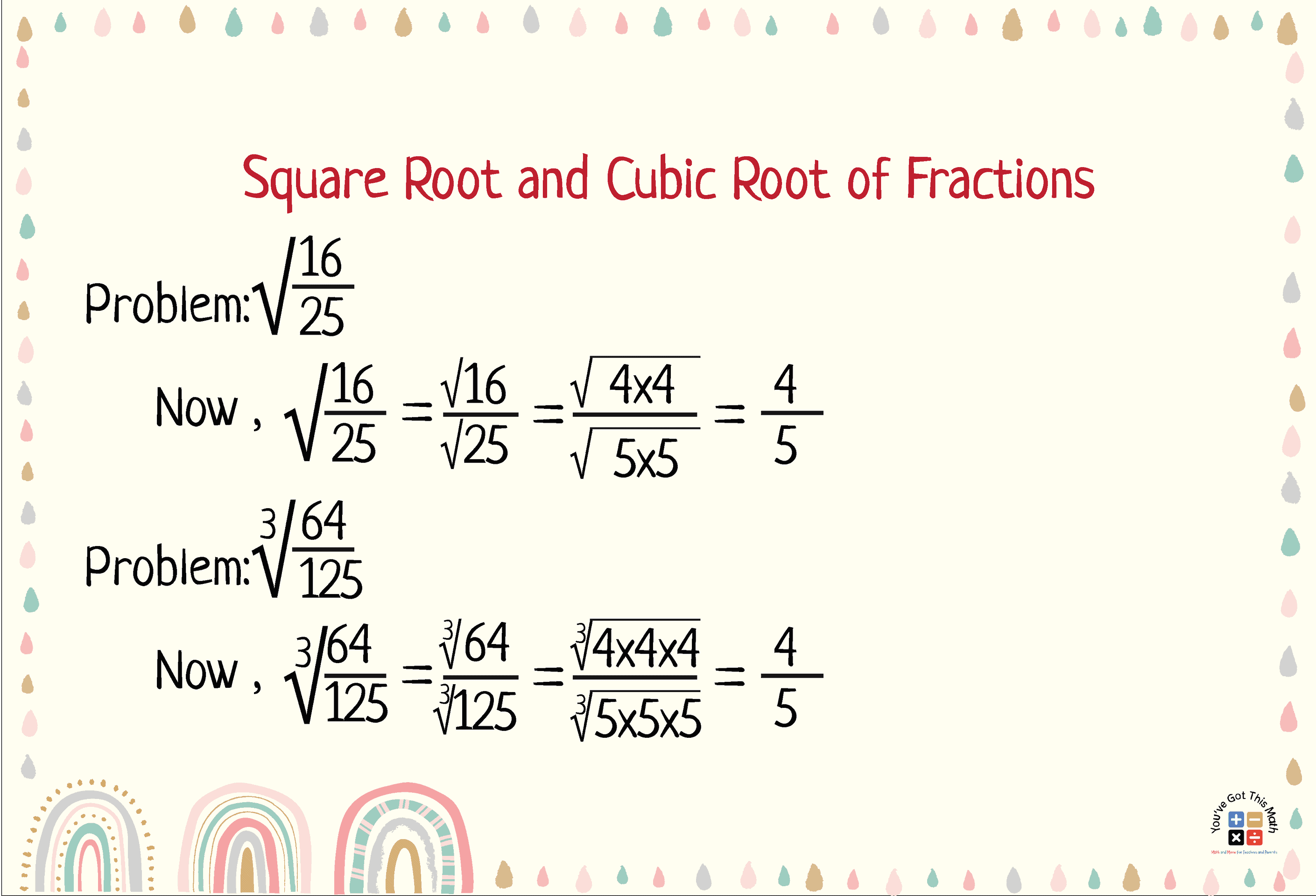10 Square Root and Cube Root Worksheet | Free Printable
In mathematics, you can square a number by multiplying the number two times and cube a number by multiplying three times. These square root and cube Root worksheets will help to visualize and understand squares, cubes, and the roots of a number. 7th or 8th graders will learn basic squares, cubes, and the roots methods and can improve their basic math skills with our free printable worksheets.
10 Square Root and Cube Root Worksheets
With the aid of these worksheets, our kids will be able to master fundamental mathematics quickly and actively. Download the following worksheets and practice.
The provided worksheet will help you to practice more examples to develop a strong number sense in square roots and cube roots. This article is especially for grade 7-8 students.
Follow the detailed instructions provided below.
Square Root and Cube Root: Easy or Hard?
In this section, we will go through some basic discussion regarding square root and cube root.
Find the Square Root of a Number
There are several methods to find the square root of a number. Here are three commonly used methods:
Method 1: Using a Calculator
- Enter the number you want to find the square root of on your calculator.
- Press the square root button.
Method 2: Long Division Method This method involves long division and is a bit more complicated, but it can be done without a calculator.
- Write the number whose square root you want to find.
- Place a bar above the number, separating it into pairs of digits starting from the right.
- Starting from the left, find the largest number whose square is less than or equal to the first pair of digits.
- Write this number above the first pair of digits, subtract the square of this number from the first pair of digits, and bring down the next pair of digits.
- Double the number above the bar and write it below the first pair of digits and to the left of the second pair of digits.
- Find the largest number whose square is less than or equal to the number you just wrote, and repeat the process until you have found the square root to the desired number of decimal places.
Method 3: Estimation Method This method involves estimating the square root by rounding the number whose square root you want to find to the nearest perfect square.
- Round the number whose square root you want to find to the nearest perfect square.
- Find the square root of the perfect square using a calculator or the long division method.
- Use the estimation to adjust the result.
Examples:
Example 1: Using a Calculator Find the square root of 144.
- Enter 144 on your calculator.
- Press the square root button.
- The result is 12.
Example 2: Long Division Method Find the square root of 625.
- Write 625.
- Place a bar above the number, separating it into pairs of digits starting from the right: 6|25.
- Starting from the left, find the largest number whose square is less than or equal to the first pair of digits (6): 2. Write this number above the first pair of digits.
- Subtract the square of 2 from the first pair of digits (6 – 4 = 2), and bring down the next pair of digits (25).
- Double the number above the bar (2 x 2 = 4) and write it below the first pair of digits and to the left of the second pair of digits: 64|25.
- Find the largest number whose square is less than or equal to the number you just wrote (25): 5. Write this number next to the 4.
- Subtract the square of 5 from 25 (25 – 25 = 0). You have found the square root.
- The square root of 625 is 25.
Example 3: Estimation Method Find the square root of 20.
- Round 20 to the nearest perfect square, which is 16.
- Find the square root of 16: square root of 16 = 4.
- Adjust the result using the estimation: (4/4) x 20 = 20/4 = 5.
- The square root of 20 is approximately 5.
Determine the Cubic Root of a Number
Moreover, there are also several methods to find the cube root of a number. Here are three commonly used methods:
Method 1: Using a Calculator
- Enter the number you want to find the cube root of on your calculator.
- Press the cube root button.
Method 2: Prime Factorization Method This method involves finding the prime factors of the number and grouping them in sets of three.
- Write the number whose cube root you want to find.
- Find the prime factors of the number.
- Group the prime factors in sets of three.
- Take one factor from each group and multiply them together to get the cube root.
Method 3: Estimation Method This method involves estimating the cube root by rounding the number whose cube root you want to find to the nearest perfect cube.
- Round the number whose cube root you want to find to the nearest perfect cube.
- Find the cube root of the perfect cube using a calculator or the prime factorization method.
- Use the estimation to adjust the result.
Examples:
Example 1: Using a Calculator Find the cube root of 125.
- Enter 125 on your calculator.
- Press the cube root button.
- The result is 5.
Example 2: Prime Factorization Method Find the cube root of 216.
- Write 216.
- Find the prime factors of 216: 2 x 2 x 2 x 3 x 3 x 3.
- Group the factors in sets of three: (2 x 2 x 2) and (3 x 3 x 3).
- Take one factor from each group and multiply them together: 2 x 3 = 6.
- The cube root of 216 is 6.
Example 3: Estimation Method Find the cube root of 37.
- Round 37 to the nearest perfect cube, which is 27.
- Find the cube root of 27: cube root of 27 = 3.
- Adjust the result using the estimation: (3/3) x 37 = 37.
- The cube root of 37 is approximately 3.3.
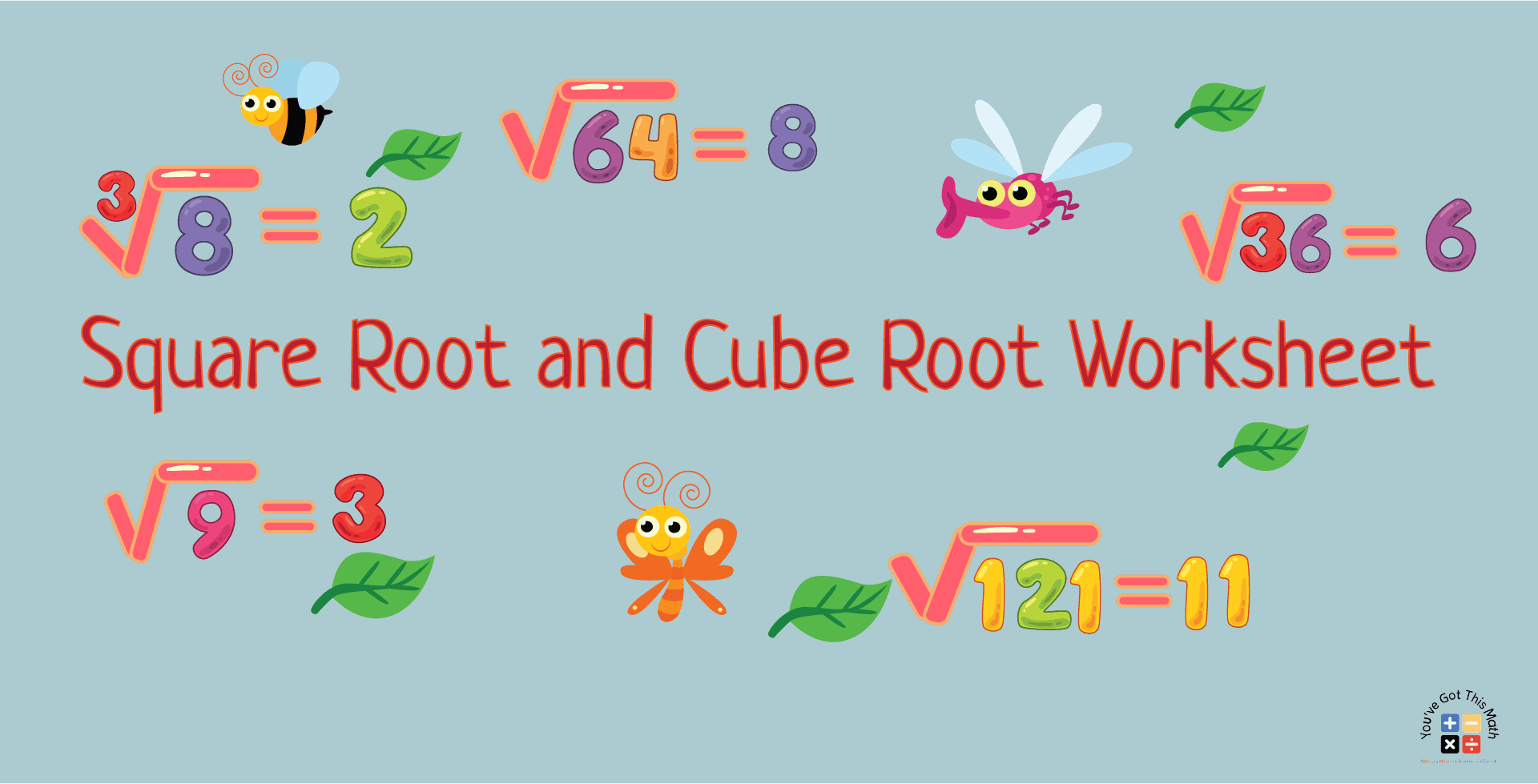
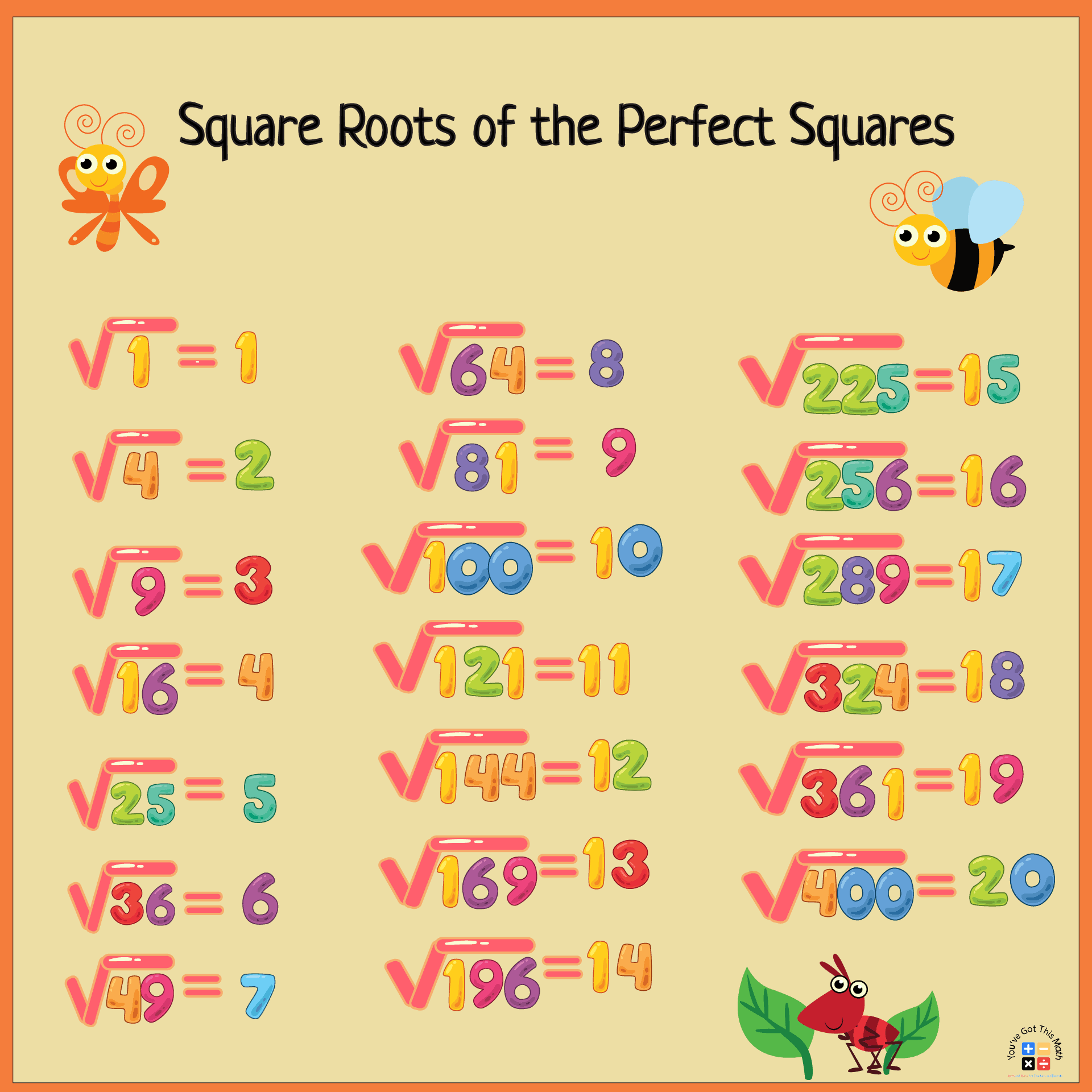
Square Roots of the Perfect Square
Here you will get square roots of some perfect squares.
Cube Roots of the Perfect Cube
Here, I will provide cube roots of some perfect cubes.
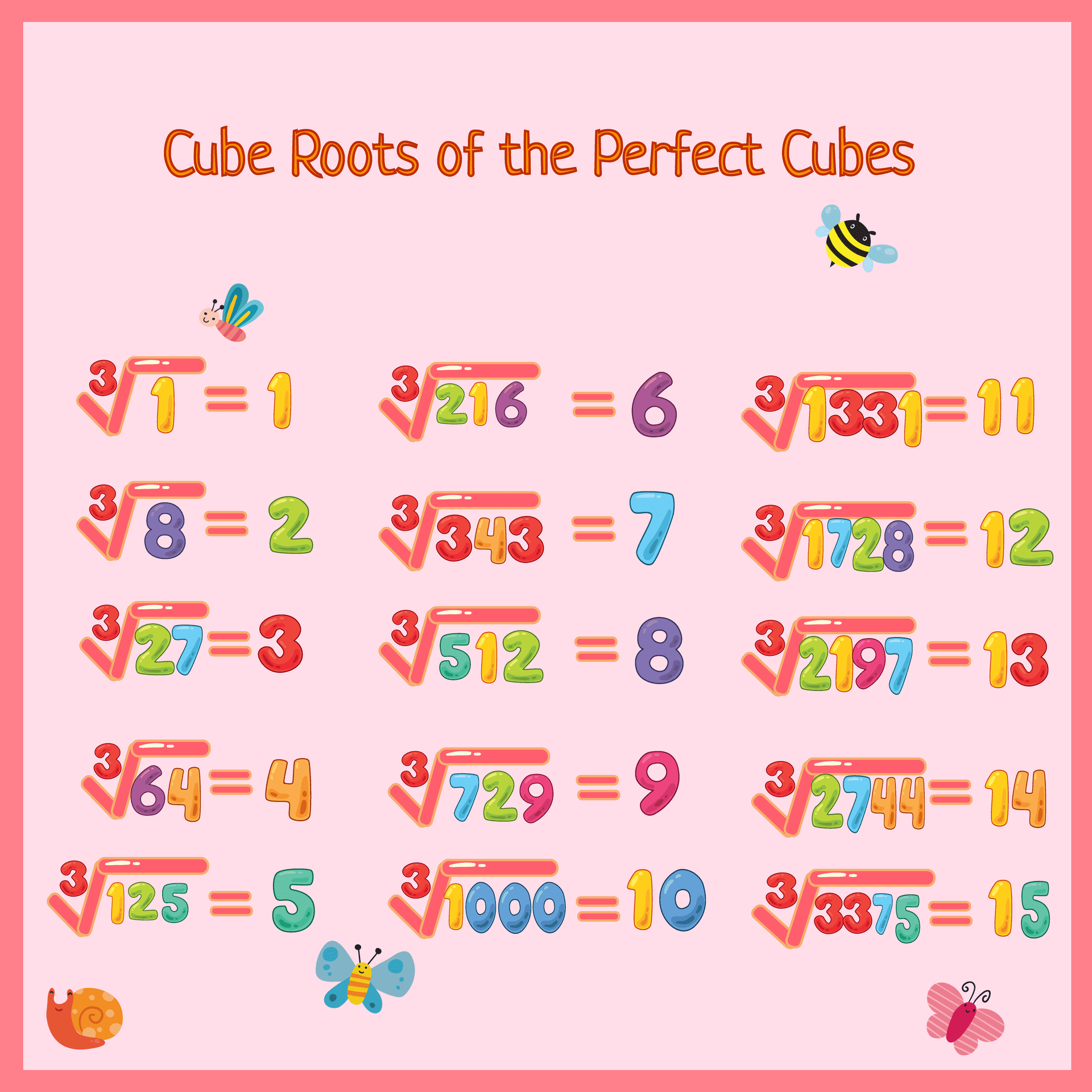
10 Activities for Square Root and Cube Root Worksheet
Get in the fun activities, download the practice worksheets, and practice more to develop the mathematic skills of your students.
Find Square Roots of Numbers
Students from 7th or 8th grades can easily solve the problems. Here, all you need to do is to find the square root of the numbers. You can use any of the three methods mentioned earlier in this article. Moreover, you can use the square root chart also.
Find Cubic Roots of Numbers
Here, you need to find the cubic roots of the given numbers. Any of the three approaches described earlier in this article can be used. The cubic root chart can also be used to determine the cubic root of a perfect cube integer. Use the worksheet to practice finding the cubic root.
Count the Dots and Get the Squares
This is a funny activity. Here you need to count the dots and then find the squares and cubs of the number of dots. For example- if you find 2 dots, you will square it to get square 4 and cube it to get cube 8.
Match the Squares and Cubes of Numbers
Here you need to match the square or cube values of some numbers given in the left column. The square and cubic values are given in the right columns. Find the squares and the cubes of the numbers given in the right column.
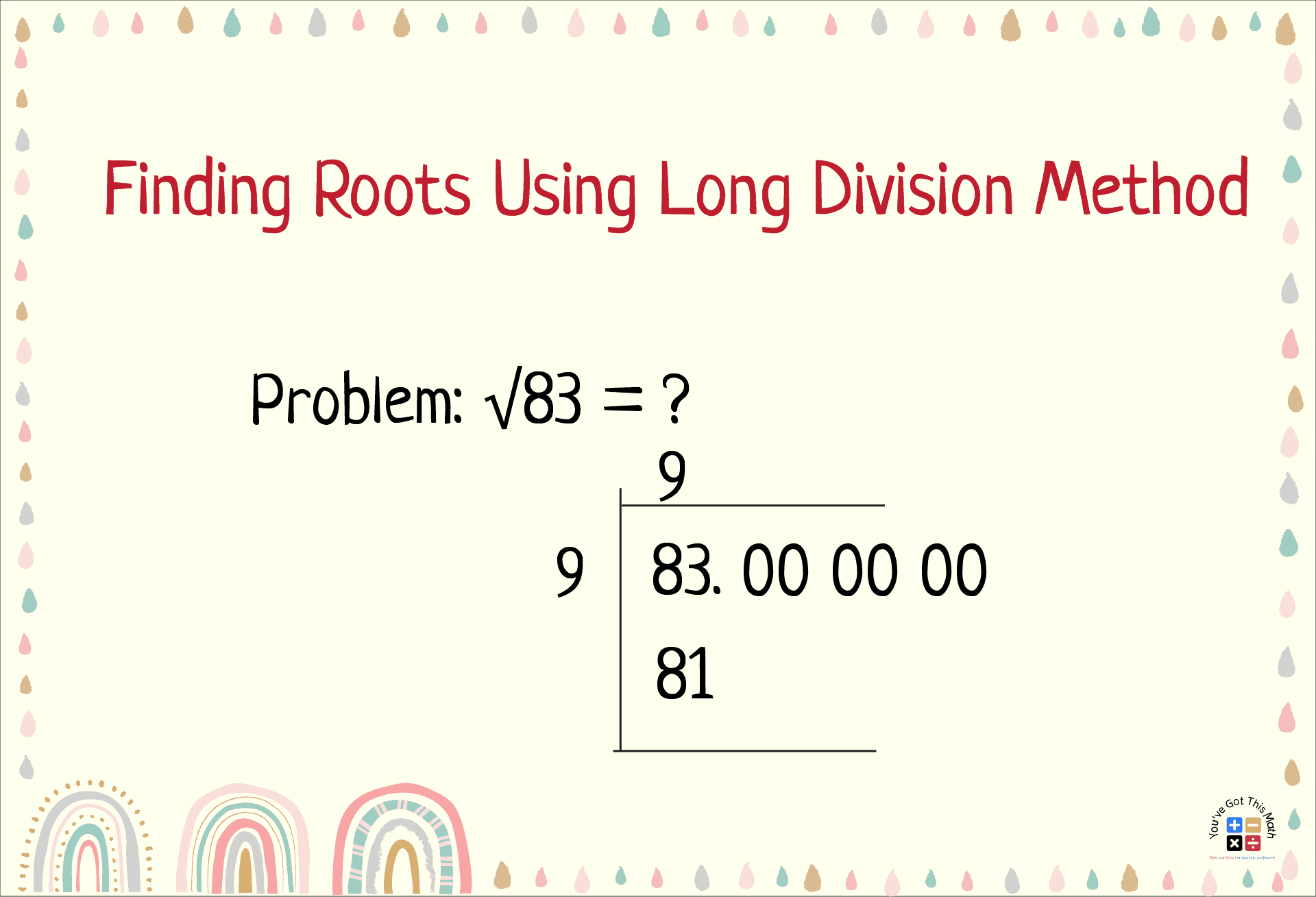
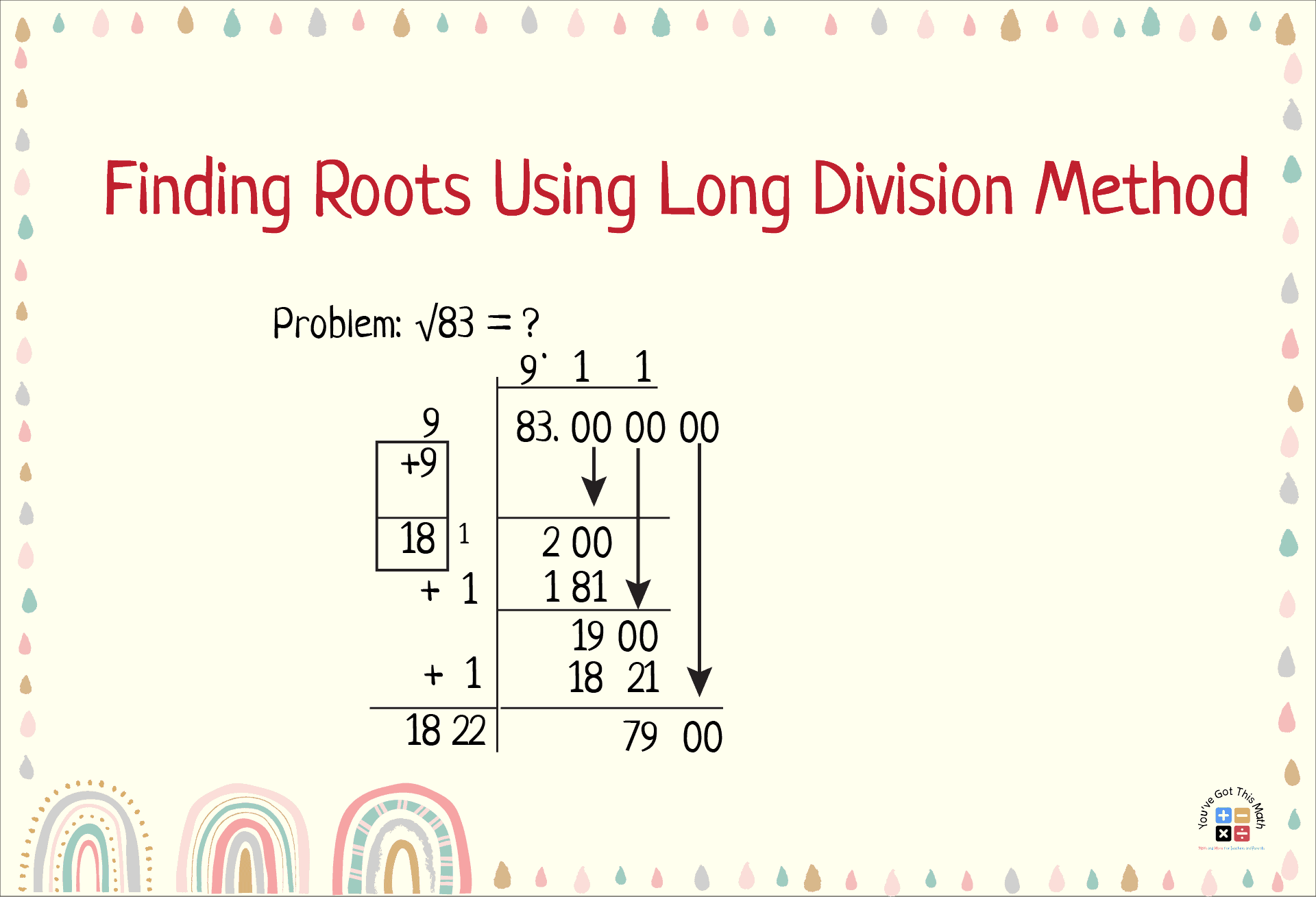
Finding Roots Using Long Division Method
This is a basic method to find a square root of a number. Follow the steps one by one to get roots. Steps:
- Let’s take 83 as a number to get the root.
- As you know from the square root chart, 83 is not a perfect square number. So, the root of the number 83 will be in the decimal point.
- Group the numbers with two digits.
- Now, place the nearest square number under 83. Here the nearest square number is 81. As 81 is the square of 9, place 9 over the top bar and left side of the vertical bar.
- Now subtract 81 from 83 and get the result 2.
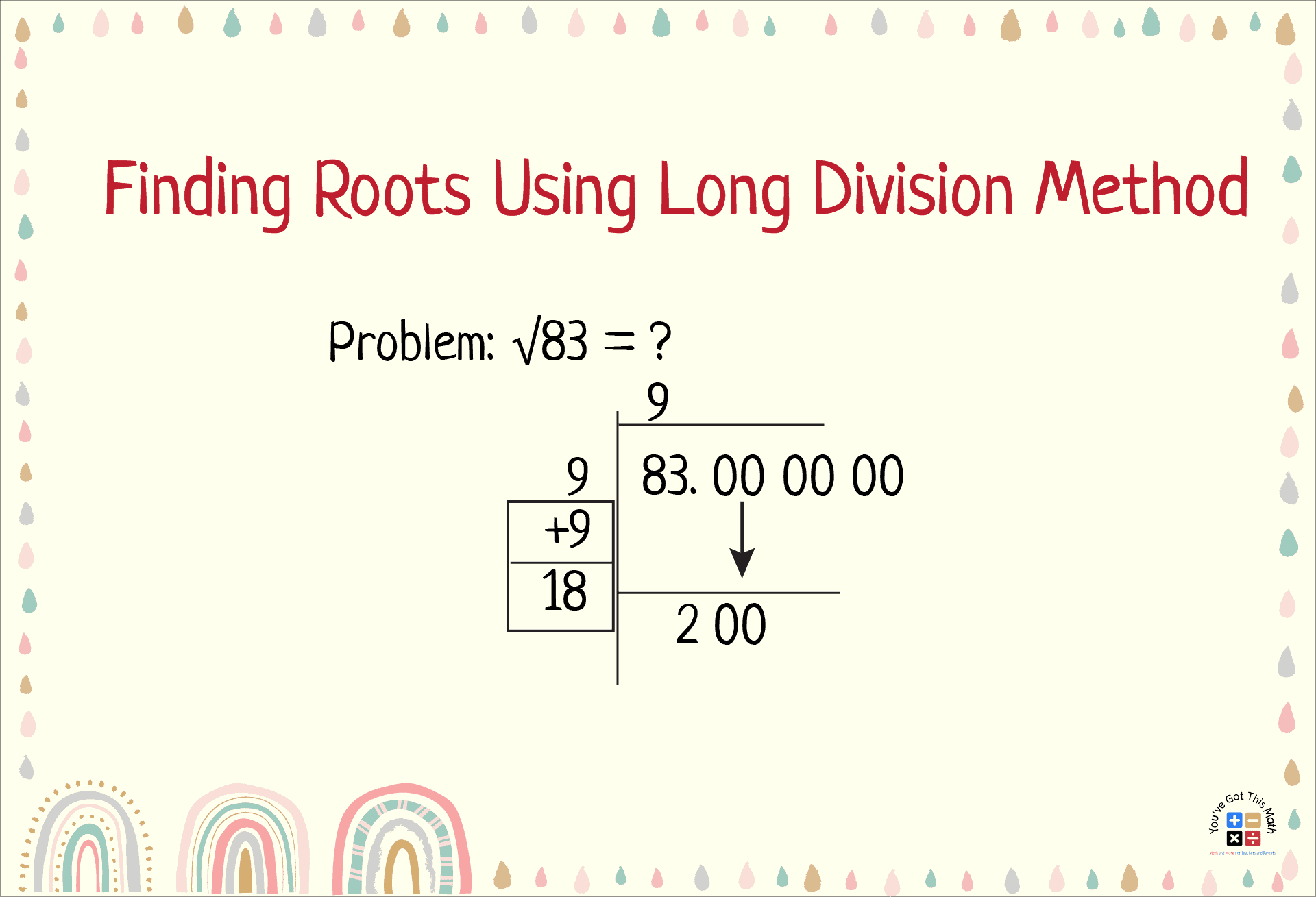
- Place the double zero at the right side of 2.
- Place a decimal point at the right side of 9 over the top bar.
- Then add the same number you place at the left side of the left vertical bar. Here, add 9 with 9 and get 18.
- You have got 200 and 18 from the last 3 steps.
- Now place the smallest number on the right side of 18 and multiply the number with that number. Here, the smallest number is 1. Place the number on the right side of the number 18 and you will get 181. Multiply 181 with 1 and subtract it from 200 and get 19.
- Then place another couple of zeros with 19.
- Add the same number 1 with 181 and get 182.
- By this process, you can get the square root of any decimal numbers or the numbers not perfectly squared.
Now practice the procedure from the following worksheet.
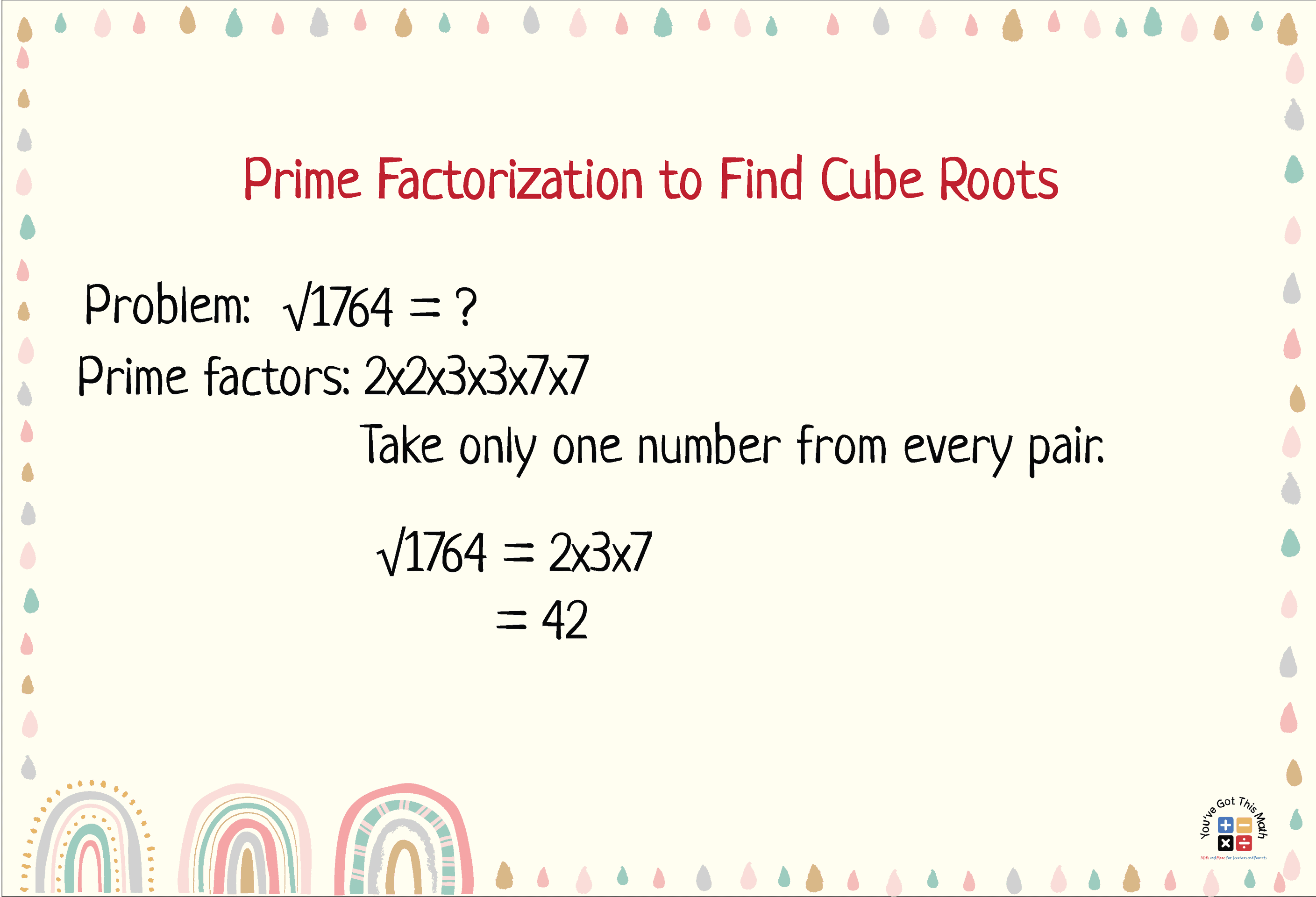
Use Prime Factorization to Find Cube Roots
When a given number is a perfect square, one can use the prime factorization method to determine its square root.
In the beginning, determine the prime factors of the given number.
The second step is to create groups of related factors.
The third step is selecting one element from each pair in the product of prime factors.
For example, you need to get a square root of 1764.
Now practice the procedure from the following worksheet.
Get the Square Root and Cubic Root of Fractions
This is a simple activity. If the numerator and denominators are perfect square and cube, just get the roots of them and you will get the square root and cubic root of fractions. If the numbers in the fractions are not perfect square and cube, use any of the methods to get square roots and cubic roots.
Now practice the procedure from the following worksheet.
Square & Cubic Roots with Addition and Subtraction
Here, you need to add first and then root the sum. You will get 100 after summation. Then square root the number 100. You will get 10. Here, you need to get the cubic root first and then subtract the numbers. You will get 5 and 3 from the cubic root and get 2 after subtraction.
Square & Cubic Roots with Multiplication and Division
This activity is similar to the previous one. Here, you need to multiply or divide numbers before or after cubic roots. For Example, First, multiply 81 and 9. You will get 729. After square root, you will get 27.
Square & Cubic Roots Word Problems
Here, some square and cubic root word problems are given. You can practice the problems more from the square root and cube root worksheets. Use any of the previous methods to get the roots.
Download the Worksheet PDF
Download the following combined worksheet and practice more with your students.
So today, we’ve discussed square root and cube root worksheets using the concepts of finding square roots of a number, finding cubic roots of a number, counting the dots, matching the squares and cubes of a number, finding roots using long division methods, using prime factorization to find a cubic root, getting the square root and cubic root of a fraction, square and cubic root with addition and subtraction, square and cube roots with multiplication and division, and word problems. Download our free worksheets, and after Practicing these worksheets, students will surely improve their mathematical skills and have a better understanding of mathematical senses.
You have got this!!

Hi there! This is Souptik Roy, a graduate of the Bangladesh University of Engineering and Technology, working as a Content Developer for the You Have Got This Math project of SOFTEKO. I am a person with a curious and creative mind. After finishing my Engineering degree, I want to explore different fields. This is why I am working here as a content developer. I have a massive interest in creative content writing. When I find that someone can learn something from my articles, this gives a lot of inspiration. hopefully, you will find interest in my article, if you have a child and want to teach them math with fun.